Steinmetz Solids

Steinmetz solids were discovered by the American-German engineer Charles Steinmetz who was active during the end of 18^th century and early 19^th century.

A Steinmetz solid is the intersection of two or three cylinders, whose axes of these cylinders stand perpendicular to each other. These solids are also called bicylinder or tricylinder, respectively.

These are the only possible Steinmetz solids in three dimensions, since one can only construct up to three axes that are perpendicular to each other. In spaces of higher dimension n however, Steinmetz solids of up to n cylinders are possible.

Rolling Steinmetz solids

Steinmetz solids behave interestingly when rolling. They can only roll in the directions following the roundings of the constituent cylinders.  

A rolling object often (but not necessarily, e.g. the oloid) possesses a smooth rotational symmetry. Cylinders are rotationally symmetric with respect to the main axis.

In mathematics, we often describe symmetries with so called groups. A completely rotationally symmetric object is invariant under the action of the group SO(3), while the subgroup SO(2) describes rotational symmetry with respect to an axis. A cylinder is thus invariant under the action of SO(2). A more rigorous treatment shows that this invariance implies that the solid can roll with respect to the axis which is fixed by this action.

It is interesting to note that the intersection of two rotationally symmetric solids need not be rotationally symmetric. For example, the bicylinder is not smoothly rotationally symmetric: If we rotate it by a small angle, it will not look the same.

It does however possess two discrete symmetries: if we rotate it by 180°, along one of the axes, it will look exactly the same. These symmetries correspond to the rolling directions.

Using the language of groups, these are two different Z₂-symmetries. The tricylinder even possesses three discrete Z₄-symmetries, corresponding to rotations of 90 degrees.

Generalized Steinmetz solids

We can generalize the concept of Steinmetz solids, by omitting the requirement that the cylinders stand perpendicular to each other. For example, one can arrange four cylinders forming the tetrahedral angle of ~109°.

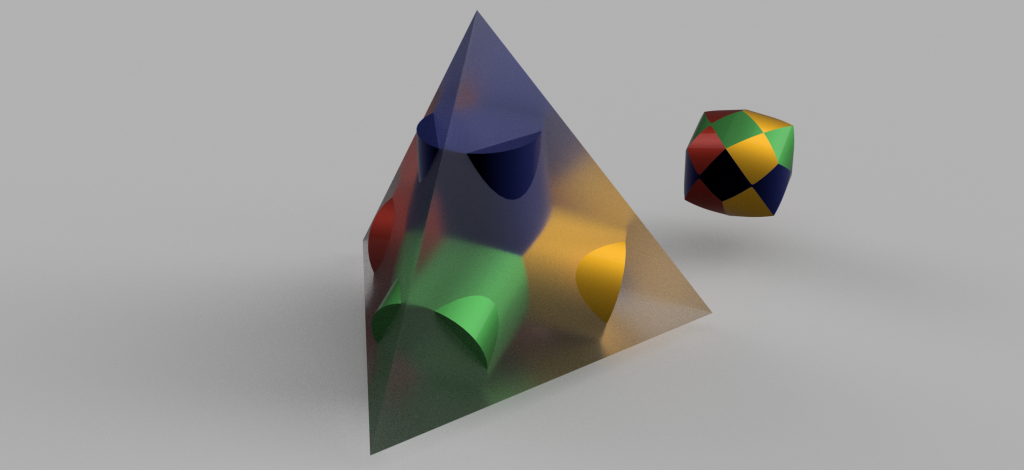
It is palpable that the intersection of n cylinders thus enables the solid to roll back and forth in n directions. In fact, it can be shown that the volume of the intersection converges to the volume of sphere. By increasing the number n of cylinders, the number of symmetries increases to n. At the same time, the symmetries get smaller repetition angles, i.e. the number m of the symmetry group Z_m is growing, e.g. the tetracylinder possesses four Z₆ symmetries.

Introduction to the concept of duality

As we saw above, we could arrange four cylinders such that their axes are perpendicular to the surfaces of a tetraheder.

Generalizing this principle by aligning the cylinders perpendicular to the surfaces of other polyhedra, one obtains similar intersections.

For example, we can connect the two opposing surfaces of a cube, and obtain the tricylinder. In fact, one reckons that the tricylinder can be obtained from two different polyhedra, namely the cube (= hexahedron) and the tetrahedron by connecting the opposing corners. This example is hence declaratory of the concept of duality:

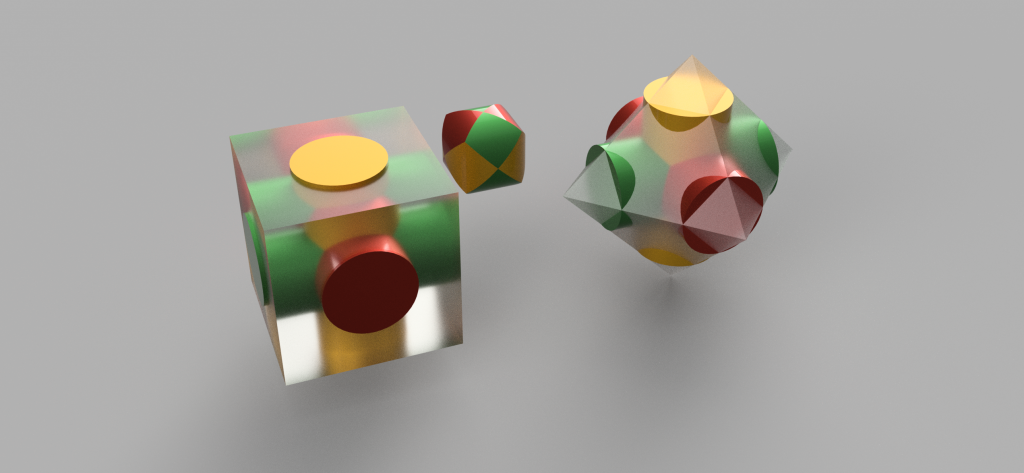
When assigning a corner to a surface and vice versa one obtains the corresponding dual solid.

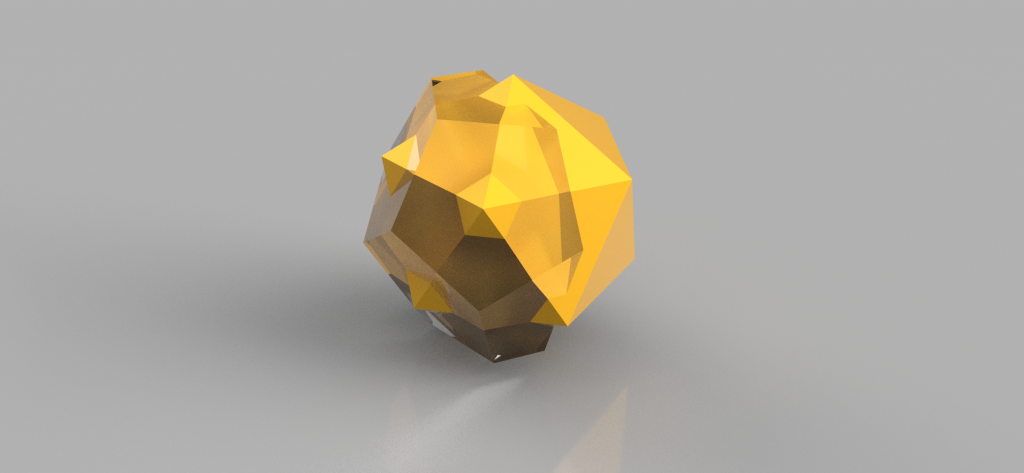
The concept of duality is tremendously important in Mathematics. Many mathematical objects have a corresponding dual object. Very often, their relation enables the scientist to discover properties of the original object by investigating its dual.

Examples of duality include:

• Logical propositions can be negated. The negated proposition is the dual to the original proposition. It is often easier to disprove the dual proposition than to prove the initial proposition.
• To every vector space, there exists a dual vector space, which is the space of all functionals on the original vector space.
• A graph consists of vertices which are connected by edges. The dual graph can be obtained by assigning a vertex to an edge and vice versa.

More about Steinmetz solids (outside of HITS website):

• Interactive program for intersections of cylinders: https://demonstrations.wolfram.com/IntersectingCylinders/
• Computation of volume and surface: http://mathworld.wolfram.com/SteinmetzSolid.html
• Intersection of randomly oriented intersecting cylinders: http://paulbourke.net/geometry/cylinders/