Uncertainty Quantification for blood pump simulation
Aims & objectives
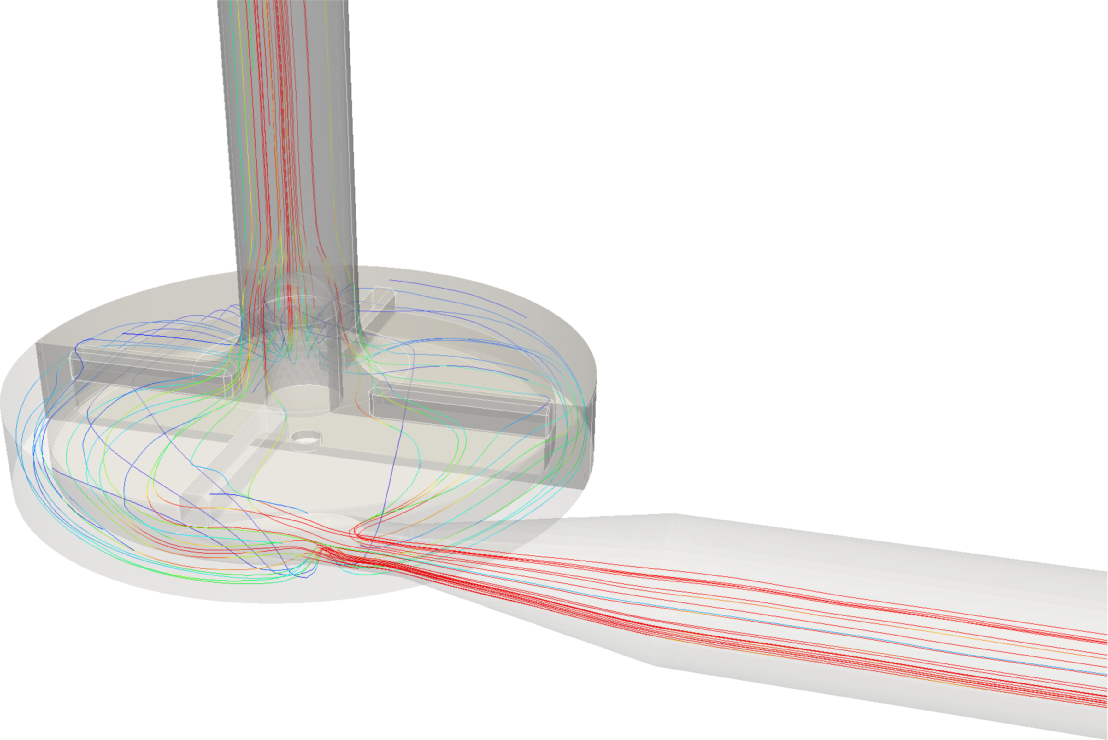
The ventricular assist device (VAD) – blood pump – is one of the most important cardiac therapeutic instruments in the last two decades, more than 23 million people are suffering from heart failure (HF) worldwide. Meanwhile, the centrifugal pump is then the most comm concept for transcutaneous and implantable VADs which support patients for augment or replace the function of failing heart.
Nowadays, computational fluid dynamics (CFD) plays an important role for modeling blood flow in complex geometries. Especially for such medical devices, many crucial information could be obtained via deterministic numerical simulations in order to quantify blood damage level such as destructive shear stresses, stagnation points, vortices, etc.
Despite the deterministic numerical models are largely and successfully introduced, but there are still many unpredictable factors which we could not have the viability to anticipate, and those uncertain aspect could lead unreliable situations which are not attained via classical simulations. Therefore, uncertainty quantification (UQ) is crucial in numerical simulations. In this project, we develop appropriate numerical solution methods to quantify the effect of uncertainty propagation in the technical device modeling the blood pump. To this end, Finite-Elements and stochastic Galerkin projection methods based on Polynomial Chaos are combined and ported to supercomputing large scale platforms.
Research topics
- Multiple reference frame methods
- Sliding mesh
- Stochastic Galerkin projection
- Multilevel solvers
People
- Chen Song
- Michael Schick
- Vincent Heuveline
Partners
Engineering Mathematics and Computing Lab (EMCL) at IWR, Heidelberg University